Displaced Cadential Six-Four Chords

Gabriel Fankhauser

Abstract

Building on research into the efficacy of inverted cadential six-four chords, this article proposes that some unconventional harmonies function as cadential six-fours. Considering a cadential six-four chord’s syntactical role more than its surface harmony or voice leading, this article identifies remarkable treatments that defy traditional analysis. Some examples of chromatically displaced cadential six-four chords seem harmonically strange yet continue to support underlying conventional function. As a result, a conflict forms between a chord’s grammatical clarity or syntax (the function of the chord in a progression) and its morphology (the chord’s pitch content and vertical arrangement). Analysis of excerpts from diverse styles—including examples from Wagner, Brahms, the Beatles, and the Eagles—illustrates how alterations of the cadential six-four chord help maintain its relevance in post-Classical music. More notable displacement in music by Liszt, Prokofiev, and Shostakovich exhibit idiomatic usage that intertwines conventional syntax and structure with innovative grammar and expression.

View PDF
Return to Volume 37

Keywords and Phrases: cadential six-four chords, harmonic function, chromatic harmony, enharmonicism, syntax, hypermeter, cliché, Wagner, Brahms, Liszt, Shostakovich, Prokofiev

1. The Cadential Six-Four Chord Controversy

Few basic topics in music theory are as controversial as the cadential six-four chord. Even the question of whether the cadential six-four chord stems from dominant or tonic harmony seems to remain as heated today as it was 250 years ago, when Johann Kirnberger responded to what he already considered an “eternal dispute” by asserting definitively that the bass of the cadential six-four chord is the root and that the chord contains linear, dissonant embellishments of the dominant harmony. In his 1773 treatise, he proclaimed:

The real root of this dissonant six-four chord is the bass note. Since the sixth as well as the fourth in this chord are non-essential dissonances, they can neither be introduced freely, nor be doubled. . . And thus an end would finally be put to the eternal dispute . . . about which so many written wars have been waged with unspeakable bitterness without anything having been settled (Beach and Thym 1979, 176).

Kirnberger’s narrow eighteenth-century definition is challenged throughout the musical literature, in which examples double fourths or “freely” introduce the sixth or fourth. Still, his interpretation continues to be embraced today. While modern pedagogy in North America tends to clarify that the cadential six-four chord functions more as a dominant than as a tonic, the chord is often introduced as a second-inversion tonic triad—a “one six-four.” Part of the justification is heuristic. Inverted triads require no contextual analysis and are therefore more easily grasped than suspensions or other linear embellishments.¹

The conflict of tonic-vs.-dominant function has continued for centuries. The simpler, vertical, “second inversion” tonic analysis (I$$^{6}_{4}$$–V) may be associated with Wilhelm Friedrich Marpurg and Abbé Vogler’s eighteenth-century harmonic emphasis on chords, largely in isolation, while the more linear and contextual analysis (V$$^{6–5}_{4–3}$$) maintains a Viennese tradition including the mid-nineteenth- and early twentieth-century theories of Simon Sechter and Heinrich Schenker.² A significant part of the problem lies with the meaning or application of Roman numeral labels. Do they signify chords (pitch content) or function (in context)? While scholarship today understandably favors the latter interpretation, an analytical approach adopted in this article may pull the preference uncomfortably toward the former—toward a simplistic vertical description, despite an understood, underlying dominant function.³ 

The linear, double suspension or appoggiatura analysis applies most readily to music composed in the late eighteenth and early nineteenth century. The behavior of the sixth and fourth as suspensions can be demonstrated by isolating and resolving each interval above the bass: compare V$$^{6–5}_{4–3}$$ to the isolated suspensions V$$^{6–5}$$ and V$$^{4–3}$$. Resolution of pre-dominant harmonies to the cadential six-four bolsters its function as an embellished dominant, rather than an inverted tonic. 

In opposition to viewing the six-four figures as linear embellishments, interpretation of the cadential six-four as a second inversion tonic can be demonstrated by replacing any V$$^{6–5}_{4–3}$$ (or I$$^{6}_{4}$$–V) with root-position I–V. Even when the I–V replacement follows strong pre-dominant harmony—like V$$^{6}_{5}$$/V, vii$$^{\circ7}$$/V, or Gr$$^{+6}$$—the momentary root-position tonic causes little interference in the harmonic progression toward the cadential dominant.⁴

Some analysts may maintain that this preservation of function from a strong predominant to the dominant demonstrates the underlying dominant function that is preserved behind the apparent tonic harmony—that the functional root continues to be $$\hat{5}$$ even if the bass is $$\hat{3}$$ or $$\hat{1}$$. Their arguments, to be clarified below, offer an important step toward appreciating both the robust essence of the cadential six-four chord as dominant in function as well as the chord’s surprising variability on the surface. 

In music from the mid-nineteenth century and later, the cadential six-four may have become more harmonically conceived. In some examples, the chord seems less dependent on adjacent harmonies—as from pre-dominant chords with pitches suspending and then resolving into the cadential dominant—and more harmonically independent. That is, the cadential six-four became an increasingly separable term in the vocabulary of Western tonal harmony. Complex examples later in this article show how the harmonic conception and independence of cadential six-four chords developed into the twentieth century. 

This article considers surface flexibility of the cadential six-four through what may be a natural development in the maturation of the singular harmony. Adopting an alternative, rhetorical perspective may circumscribe and inform the perennial tonic-vs.-dominant controversy by highlighting how the cadential six-four is defined more by its context in a cadential progression than by either its isolated harmonic components or its melodic-contrapuntal connections.

Motivated in part by the heuristic efficiency of identifying the cadential six-four chord as a “second-inversion tonic triad,” Leonard Meyer (2000/1992) views the chord as part of a conventional gesture that is best represented as tonic in function. While he “grants the plausibility” of the interpretation of a double appoggiatura over dominant harmony, he “finds it more convenient . . . to cling to the more traditional (conventional?) interpretation and symbology” (230, fn. 9, sic). Using a somewhat narrow definition, Meyer searched for examples throughout the Norton Scores and found that usage of the cadential six-four progression reached a sharp but short-lived peak in the Classic era. His findings are summarized in Figure 1. Meyer’s data show that usage increased most notably with the music of Domenico Scarlatti and then waned, most notably with the music of Hector Berlioz, who, Meyer says, rejected the six-four progression as the “epitome of conventionality” (248).⁵ Romantic composers may have avoided the chord, because, as Meyer adds, “the very sonic salience that made the cadential six-four progression such a forceful signal [of closure] made it seem routine and commonplace.” For composers aiming to avoid tonal formulas and predictability, the cadential six-four became outdated or obsolete.

If the cadential six-four generally became viewed as an unappealing conventional gesture, then a decline in usage would not be surprising. However, compositional devices that are viewed as clichés can, after some period of disuse, sow seeds for revival, often with fresh approaches. Rhetorical clichés result when expressions that were novel at one time suffer from overuse, either losing their original meaning or becoming conspicuously predictable. The meaning of a cliché derives not solely from the words or parts themselves but more from a collective complex of meanings tied to its usage. English clichés like “the tip of the iceberg” or “thinking outside the box” are often considered signs of sophomoric writing to be avoided in scholarship, but an exception arises when an author purposefully or poetically uses the phrase to allude to a second or deeper meaning—a subtle, intentionally rhetorical usage. 

Like literary clichés, cadential six-four chords possess predictability and potential for overuse that may have deterred some nineteenth-century composers. While the strong syntactic power of the cadential six-four to initiate closure may have led to its decline in the late-nineteenth century—a period that saw a decline in well-defined closure and formal divisions—the clarity and simplicity of the chord’s grammatical function may have conversely provided means for subsequent composers to express an individual and innovative harmonic voice. As a cliché, the cadential six-four could be viewed as an inhibitor of musical originality, but with careful treatment a twisting of the cliché could afford composers fresh means for approaching a cadence. A distinctly modified cadential six-four may retain its cadential function as a rhetorical signal for closure, borrowing from Classical syntax, yet be altered in a way that transforms the cliché into novelty. Composers today continue to borrow familiar materials from the past in a more individualized, modern context.

Straddling the threshold between harmonic tradition and innovation, this study supports two main ideas concerning the cadential six-four. The first is that, after conventional use of the cadential six-four reached its peak late in the Classic era, later approaches significantly modified the harmony on the surface while retaining the chord’s underlying rhetorical, cadential function. The second idea is that analysis of those modifications informs our understanding of the cadential six-four chord as separable into two components: its contextual usage, or syntax, and its outward appearance, or morphology. Different musical examples may alter one component more than the other. On one hand, the function of an otherwise conventional cadential six-four may be undermined by its treatment, as by weak-beat placement or by irregular resolution, to the point that its cadential function becomes questionable. On the other hand, examples may retain conventional treatment, particularly in accentuation and resolution, while altering the chord’s outward appearance or pitch content. It is this latter, morphological alteration that lies at the center of this study.⁶ Focus will be on vertical (pitch) displacement of the cadential six-four, but first consider one example of horizontal (rhythmic) displacement. 

Placement of the cadential six-four on a strong beat in relation to its resolution to dominant five-three is a defining characteristic of the cadential six-four chord. The inherent contradiction of a weak-beat cadential six-four chord makes them rare.⁷ Wagner’s “Song of the Evening Star” from Tannhäuser presents an example (Example 1). In m. 10, the six-four enters on a weak, second hyperbeat, followed by a resolution on the stronger downbeat of m. 11. The only way to hear the six-four fall on a strong hyperbeat would be to hear the opening measure as an anacrusis. The third harmony, an apparent $$\flat$$III$$^{6}$$, exhibits a weaker association with a cadential six-four, perhaps as a modified V$$^{6–5}$$. We will return to this example after examining other displacements of the cadential six-four.

More remarkable deviations from conventional cadential six-four chords lie not with accentuation but with pitch content and arrangements.⁸ The following section examines how the chord itself can be modified, starting with inversion.

2. Inverted Cadential Six-Four Chords

Placing the cadential six-four chord in inversion alters its outward appearance and increases its resemblance to a consonant tonic triad. Finding support in Schenker’s earlier, unpublished version of Der freie Satz, William Rothstein (2006, 268–272) observes how an apparent root-position or first-inversion tonic may function as a cadential six-four. Example 2 shows his analysis of a passage from Mozart’s Horn Concerto in D, K. 412. While the original voice leading shown in (a) has an F$$\sharp$$ in the bass ($$\hat{3}$$) in m. 28, Rothstein shows (in b) how A ($$\hat{5}$$) remains as an imagined or underlying Stufe. Mozart’s inversion of the cadential six-four is required to resolve the preceding (surface) V$$^{4}_{2}$$ and to avoid parallel octaves with the melody’s local ascent from G4 to A4, $$\hat{4}$$ to $$\hat{5}$$.

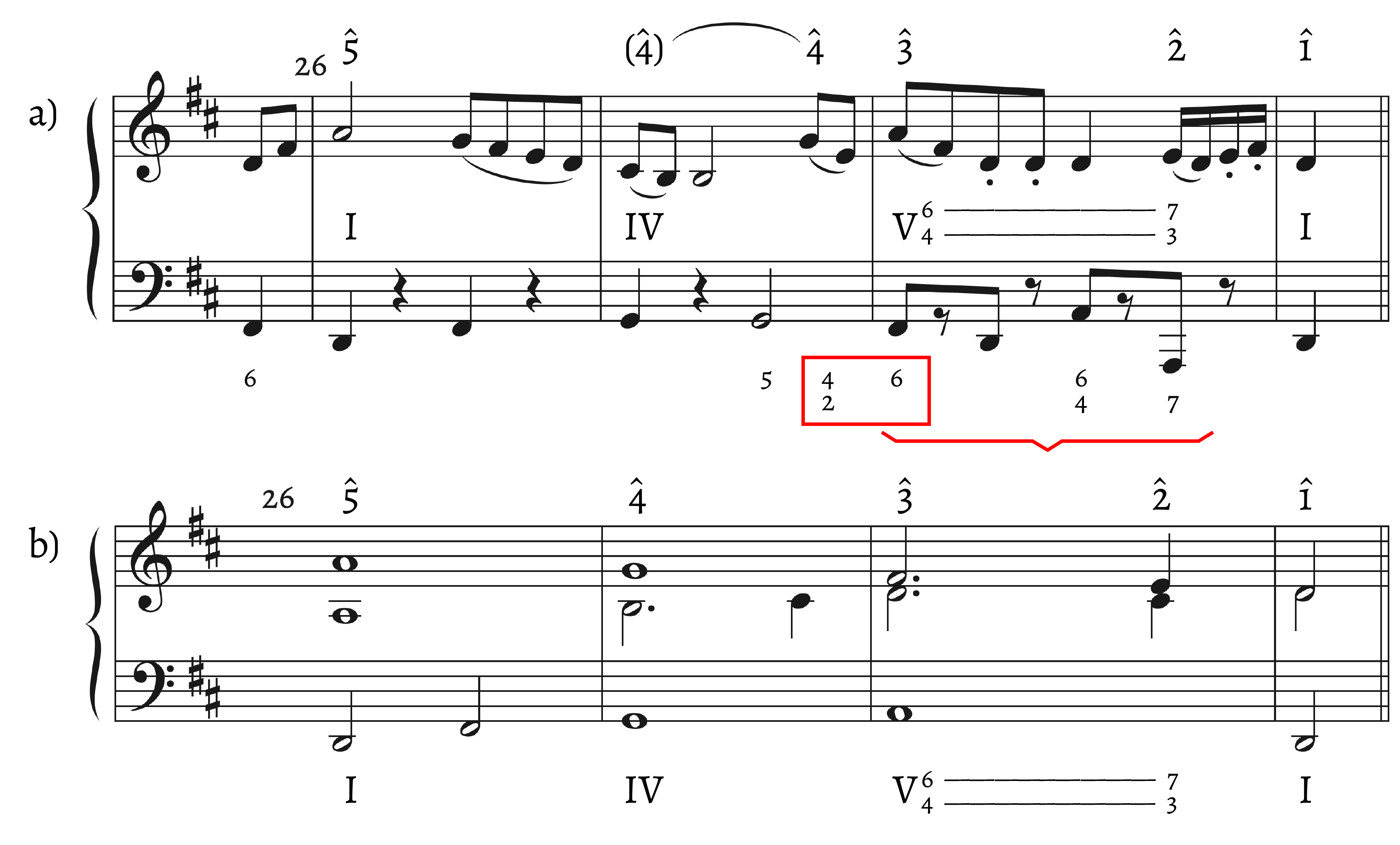
The concept of inverted six-four chords introduces an analytical challenge. First, it implies that a suspended note—namely $$\hat{3}$$ or $$\hat{1}$$ of a cadential six-four—is placed in the bass and then skips up into the following root-position dominant ($$\hat{5}$$). Rothstein’s omission of Roman numerals below his analysis avoids confusing the chord’s underlying dominant function on one hand—shown between the staves in (a) and below the normalization in (b)—and the apparent inverted tonic triad on the other, indicated by the figured bass 6 below the staves. 

For a surface Roman numeral analysis like I$$^{6}$$ to conflict with the underlying bass Stufe like $$\hat{5}$$ indicates a contradiction between two levels of analysis. Rothstein’s “V$$^{6–7}_{4–3}$$” analysis in (a) more accurately addresses harmonic function in the third measure, even if “I$$^{6}$$–$$^{5}_{3}$$–V$$^{6–7}_{4–3}$$” more accurately describes the surface harmony. Since dominant function remains an essential characteristic of the cadential six-four, it would follow that cadential six-fours should be associated with the dominant using the label “V.” The problem is that that label requires multiple levels of interpretation: the apparent I$$^{6}$$ inverts a conventional cadential six-four, which displaces the third and fifth of a dominant. Analysis of more unusual examples may benefit from using the more specific morphological or basic harmonic (vertical) description, following the heuristic of the “one six-four” (I$$^{6}_{4}$$) literal description, even if that analysis remains comparatively on the surface and in conflict with the harmony’s underlying function, which remains understood as dominant, V$$^{6–7}_{4–3}$$. Analytical examples add a bracket to couple each modified cadential six-four with the dominant. 

Timothy Culter (2009) has investigated how inverted cadential six-fours result from voice exchanges, while Eric Wen has shown how a first inversion tonic triad can serve more as a replacement for a cadential six-four chord (1999, 288). As new as these concepts may seem, however, both Cutler and David Damschroder have noted that the concept of the inverted cadential six-four can be traced to Koch’s harmony treatise of 1811, in which I and I$$^{6}$$ chords are shown to be dissonant in relation to their resolution to dominant.⁹ One benefit of their more recent studies, therefore, is to challenge modern assumptions and pedagogy regarding the identity of the cadential six-four chord and to broaden our understanding of its usage.

One notable example of an inverted cadential six-four that remains unanalyzed in the literature, as far as I know, enters near the beginning of the fourth movement of Brahms’s Symphony No. 4. The familiar opening theme of the chaconne derives from the last movement “Meine Tage in dem Leide” from Bach’s Cantata Nach dir, Herr, verlanget mich, BWV150, but, in typical Brahmsian style, alteration of the subject’s structure obscures harmonic function (Example 3). Each step of an ascending melody pushes toward the climax, which coincides with a modified cadential six-four in m. 6. What appears on the surface as a first-inversion tonic functions as an inverted cadential six-four chord, supported both by the preceding, intensifying melodic drive from $$\hat{1}$$ up to $$\hat{5}$$ and by the harmonic drive toward the dominant via the subdominant iv$$^{6}$$ in m. 4 through V$$^{7}$$/V in m. 5.¹⁰ Gestures associated with cadential six-four resolutions remain present here as well but inverted: the octave drop from a high $$\hat{5}$$ to a lower $$\hat{5}$$ typical of the bass falls instead in the melody, while the bass descends by step, $$\hat{3}$$–$$\flat\hat{2}$$–$$\hat{1}$$. This linear descent is more typical of melodic closure, in this case reversing the opening motive in mm. 1–3 as well as the $$\hat{1}$$–$$\flat\hat{2}$$–$$\hat{3}$$ (E–F$$\natural$$–G) opening of the second movement.

The octave leap and $$\hat{3}$$–$$\flat\hat{2}$$–$$\hat{1}$$ descent alone support hearing an inverted cadential six-four in m. 6, but connections to Bach’s original Chaconne (Example 4) offer rare insight into Brahms’s thinking. In the opening of this last movement of Bach’s cantata, the bass rises $$\hat{1}$$–$$\hat{2}$$–$$\hat{3}$$–$$\hat{4}$$–$$\hat{5}$$ in mm. 1–4, followed by a $$\hat{3}$$–$$\hat{2}$$–$$\hat{1}$$ melodic descent toward the cadence. At the intersection of those lines lies the cadential six-four in m. 4. Brahms’s invertible counterpoint inverts the cadential six-four by placing $$\hat{3}$$ in the bass and $$\hat{5}$$ in the melody. The retrogressive opening three harmonies (iv$$^6$$–ii$$^{\circ6}$$–i) from the outset also hint at Brahms’s attraction to “inverting” traditional tonal syntax.

Identifying the inversion of the progression from Bach’s chaconne to Brahms’s symphony demonstrates that the inverted cadential six-four is more than a speculative interpretation of a particular harmony and more than an example of a late nineteenth-century composer’s innovative treatment of an early eighteenth-century trope. In this case, Brahms’s inverted cadential six-four derives from a specific historical example of a conventional cadential six-four, offering a rare glimpse of an innovative compositional process. 

The cadential six-four chord is commonly associated with the Western classical tradition and the previous two examples draw a direct link from Bach to Brahms, but the harmony may be found in other styles. Some rock music has been shown to adhere at least loosely to Classical harmonic syntax and to use cadential six-four chords specifically.¹¹ Consider the chorus of the Eagles’s “Heartache Tonight” (Example 5). The rising chromatic bass line increases drive toward the cadential dominant, but that dominant is displaced by root-position tonic harmony.¹² After playing $$\sharp\hat{4}$$ (C$$\sharp$$), the bass plays the tonic (G) before resolving up to the dominant (D), forming an inverted cadential six-four chord. Substitution of “I–V” in place of the cadential dominant or V$$^{6–5}_{4–3}$$ following vii$$^{\circ7}$$/V is common in American bluegrass and other popular styles.¹³

Conventional analysis interprets the $$\hat{5}$$ bass as the root of the cadential six-four, but in the inverted cadential six-fours described by Rothstein and Cutler (the apparent I or I$$^{6}$$ chords), the $$\hat{5}$$ root lies a fifth or third above the bass. In subsequent, more chromatic examples that retain six-four figures (like “$$\flat$$VI$$^{6}_{4}$$”), I will use Roman numerals to designate the fourth above the bass as the triadic root. A seeming contradiction in cadential six-four analysis stems from the difference between emphasizing harmonic function—by definition, an underlying dominant function for all cadential six-four chords—and triadic formulation, a literal description of the pitches that lie above the bass.

Countering traditional Schenkerian-Stufen theory, David Temperley (2017) argues that some contexts justify the use of Roman numerals to indicate localized harmony. His use of the label “I$$^{6}_{4}$$” applies “a concept that is often implicit in modern music theory but rarely defined explicitly: what we might call a surface-level harmony, or (hereafter) simply a harmony” (4). In any conventional example, adopting the narrow “I$$^{6}_{4}$$” label over the more functional and linear V$$^{6–5}_{4–3}$$ interpretation might seem trite, but the vertical description is more justified in examples that significantly modify the cadential six-four to create uncanny harmonic relations.

The difference between a triad’s root and its bass may seem evident enough, but Daniel Harrison (1994, 48) makes a further distinction between a root and a base. An unfortunate homonym with “bass,” a base refers not to the lowest note but a more conceptual foundation. He views the assertion that the cadential six-four is dominant in function (as in V$$^{6–5}_{4–3}$$) to mean not that $$\hat{5}$$ is the root but more precisely that it is the base. Whereas a root is determined by restacking a triad, a base is determined by the context of the key. In cadential six-four chords that are inverted to I$$^{6}$$, the lowest note ($$\hat{3}$$) is the bass. Vertical restacking of the triad determines the (surface) root ($$\hat{1}$$) simply enough, while $$\hat{5}$$ remains the functional base, a quasi-root. Using Harrison’s distinction, we may view the cadential i$$^{6}$$ in Brahms’s Symphony (Example 3, above) to present G as the bass, E as the root, and B as the base, which, while placed in an inner voice, still plays the greatest harmonic role in the context of the progression. Conventional cadential six-fours do not require this distinction, but in the inverted examples discussed earlier and in the more harmonically displaced examples that follow, equating the chord’s base—not necessarily its root or its bass—with the chord’s dominant function helps to elucidate the taxonomic problem, when a chord’s spelling conflicts with its function. If “$$\flat$$VI$$^{6}_{4}$$” were to function as a cadential six-four, for example, its surface root would be $$\flat\hat{6}$$, and its bass would be $$\flat\hat{3}$$, but its base would remain $$\hat{5}$$, an interpreted, absent functional root.¹⁴

3. Definition of the Cadential Six-Four Chord

Table 1 isolates properties of the cadential six-four. Characteristics 1–3 are essential, defining features: (1) cadential six-fours have strong metric accentuation in relation to their resolution to a dominant in the approach to the cadence—typically falling on hyperbeat 3 of a four-hyperbeat phrase, (2) they contain intervals of a sixth and fourth above the bass—forming an apparent triad with the fifth in the bass, and (3) they include pitches from the tonic triad—though these pitches may be altered chromatically. Characteristics 4–6 are better considered as common treatments than as defining characteristics. They describe the doubling of the bass, the downward resolution of the sixth and fourth (6–5 and 4–3), and the holding of the bass $$\hat{5}$$ into the cadential dominant.¹⁵ Deviations from Characteristics 4–6, while somewhat uncommon, pose no challenge to the function or identity of the chord.

Isolating these defining characteristics will help in the study of some unusual musical examples. Each defining characteristic invites a specific compositional challenge: how might an unconventional cadential six-four counter a given characteristic while preserving underlying, functional identity? Potential deviations are listed in the middle column, and a musical example for each deviation is listed to the right. Each listed example deviates in some respect from conventional usage but all satisfy two out of the three most essential criteria. Challenging criterion 3—that the cadential six-four consists of pitches from the tonic triad—lies at the heart of this study.¹⁶

To understand the theoretical origin of cadential six-four chords with non-tonic pitches and the remarkable excerpts listed to the right of criterion 3, reconsider the use of Roman numerals and Arabic numerals. Arabic numerals originally simply indicated intervals above the bass (figured bass), with little or no consideration of triadic harmony. Roman numerals were affixed significantly later, in the early nineteenth century. If certain aspects of the cadential six-four chord can be categorized as primarily contrapuntal on one hand, with voice leading and inversions denoted by figured bass, or harmonic-syntactic on the other, based on the pitches and harmonic context, then the two commonly used analytical symbols may be separated into two potential means for deviation: the apparent “I” and the “six-four” (Figure 2). The cadential six-four’s composition of pitches from the tonic triad is such an integral part of its identity that we see more flexibility with figured bass or inversions.

As Meyer suggested, the inherent predictability of the cadential six-four chord likely led some nineteenth-century composers to avoid it. Other composers, however, may have seen potential for flexibility in its harmony through complete transposition of the chord—a drastic alteration counterbalanced by rigid restriction of the figures to six-four. In this latter means of deviation, the six-four figured bass is retained while the pitch content—most efficiently represented by a Roman numeral—is varied. Transposition of the whole chord offers a greater potential for variety than inversion.

4. Harmonic Deviations in the Cadential Six-Four

A hypothetical spectrum of cadential six-four deviations—from the conventional through the contrapuntal to what may seem quite harmonically bizarre—is offered in Example 6. Compared to the conventional usage in Example 6a, the deviations in Examples 6b and 6c demonstrate contrapuntal flexibility while retaining the pitch content of the tonic triad. These are the types of deviation discussed by Rothstein and Cutler.

Examples 6d and 6e, however, present notably different alterations, which retain the intervals of a sixth and fourth of the conventional six-four while shifting the entire chord vertically. The remarkable iii$$^{6}_{4}$$ in Example 6d and even more unusual $$\flat$$I$$^{6}_{4}$$ in Example 6e are but two of the possible transpositions of the cadential six-four. These five hypothetical examples vary in their intervals or basses, but all maintain some defining characteristics as they displace the cadential V$$^{7}$$ from a strong beat in the context of a basic progression. Each example also supports the conventional $$\hat{3}$$–$$\hat{2}$$–$$\hat{1}$$ melodic gesture in the upper voice. While the surface analyses of each downbeat of m. 3 vary widely in their Roman numerals, all of the cadential six-fours in Example 6 maintain an underlying syntactical role and association with dominant function, regardless of substantial alterations on the surface.

An example of a iii$$^{6}_{4}$$ acting as a displaced cadential six-four (Example 6d, above) enters near the beginning of The Beatles’s “Julia” (Example 7, below).¹⁷ John Lennon plays two second-inversion mediants in the opening of his song. He uses a picking technique on the guitar that presents off-beat bass arpeggiations down to the fifth of the opening chords, but these initial, arpeggiating six-fours do not alter the underlying inversion. In the first two measures, the first bass note is more structural and holds the harmony to root position. The third chord alters the pattern, however, as the C$$\sharp$$ in the bass represents the fifth of a mediant triad to form a iii$$^{6}_{4}$$ substitute for a more conventional V$$^{6}$$. The $$\hat{1}$$–$$\hat{6}$$–$$\hat{7}$$ (D–B–C$$\sharp$$) bass line in the opening phrase sits uncomfortably on the leading tone until it leaves the iii$$^{6}_{4}$$ to pass back up to the tonic at the start of the next phrase. Following the return of the iii$$^{6}_{4}$$ near the end of the second phrase, a briefly inserted $$\hat{5}$$ (A) in the bass ($$\hat{1}$$ –$$\hat{6}$$ –$$\hat{7}$$ –$$\hat{5}$$ –$$\hat{1}$$) alters the chord’s harmonic role to become cadential as it resolves into a root-position dominant. The reappearance of the chord thereby carries more syntactical weight as a cadential six-four.¹⁸ This subtle redirection of the chord as initially passing or unresolved as it accompanies the word “meaningless” toward more defined syntactical closure as it accompanies the word “Julia” coincides with a stretching of the phrase rhythm as Lennon sings the name of his mother, who was killed in a car accident when he was seventeen years old. 

The end of Brahms’s Waltz in E major, op. 39, No. 2 is strikingly similar (Example 8). The cadential “iii$$^{6}_{4}$$” in m. 23 functions as an altered cadential six-four, one whose connection to tonic or dominant harmony is not immediately apparent but whose function is clarified by its context and resolution. Its entrance on a weak hyperbeat, the second measure of the phrase, and $$\hat{1}$$-to-$$\hat{7}$$ approach in the bass motion initially make the chord sound passing, as was the earlier correlating E–D$$\sharp$$ motion in m. 6: I–(V$$^{6}$$)–vi–V/V–V (not shown). The resolution of the six-four directly to the cadential dominant, however, confirms its cadential identity. In Brahms’s waltz, the iii$$^{6}_{4}$$ resembles the first half of a V$$^{6–5}$$ motion, but with the chordal third (D$$\sharp$$, $$\hat{7}$$) in the bass, which then skips down to the root (B, $$\hat{5}$$).

Unlike Lennon’s example and more like Wagner’s, the weak hypermetric placement of this six-four in relation to the cadential dominant further lessens its cadential quality. It is the simple progression’s syntax, more than the associated harmony or counterpoint, that makes the six-four chord cadential. The chord’s brief harmonic implication as a conventional cadential six-four in G$$\sharp$$ minor is fostered by the next waltz of the set, which begins with a G$$\sharp$$-minor tonic. 

Franz Liszt’s “Il penseroso” (Example 9) contains an apparent submediant cadential six-four chord. The bass in the opening phrase (mm. 1–4) completes a highly conventional progression: I–VI–IV–V–I. Each harmony after the opening tonic, however, grammatically and dramatically pushes the progression into a mid-nineteenth century chromatic idiom. The second phrase (mm. 5–8) expresses an equally conservative underlying syntax. Its morphological envelope is pushed further, however, as the modulating progression from C$$\sharp$$ minor to E minor wrestles through what sounds like a “wrong” cadential six-four chord, labeled below according to its pitch content as a cadential VI$$^{6}_{4}$$.

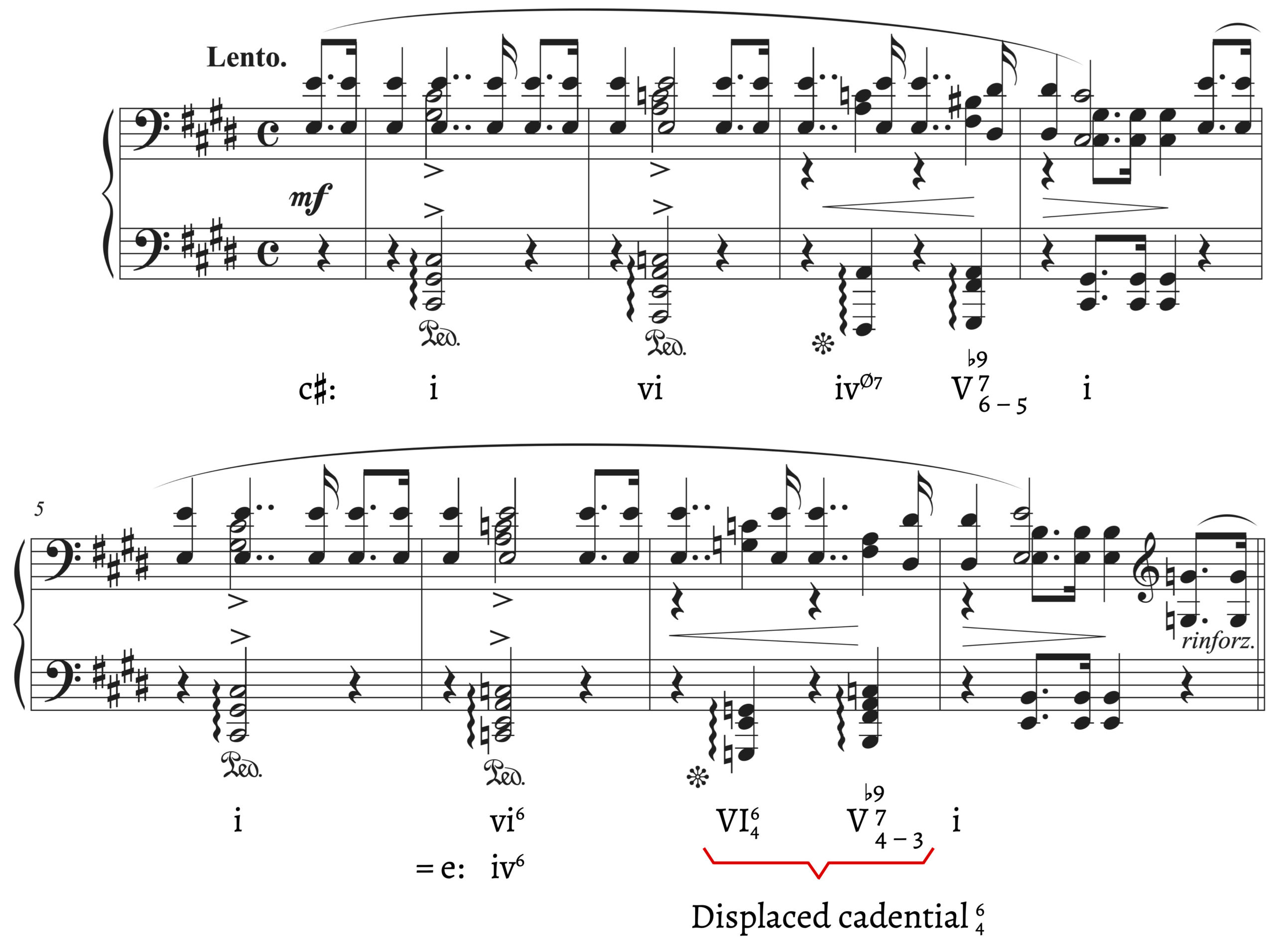
Liszt’s altered cadential six-four in this example suggests a change in nineteenth-century compositional thought. As a clear harbinger of closure, the cadential six-four provides opportunity for manipulation of expectation. Whereas original Baroque usage of the cadential six-four derives from contrapuntal displacement of dominant’s fifth and third, as through suspensions, Classical usage increased harmonic independence. As a strong signal for closure, the cadential six-four saw increased use in common-practice tonality, placing more significance on the chord as a discrete, separable harmonic entity. Increased emphasis on the cadential six-four’s vertical dimension invites expansion not merely through prolongation, as in a cadenza, but of the shape of the chord itself. 

In contrast to the largely linear Baroque usage and even to the more harmonic Classical usage, the six-four in Liszt’s 1839 example, while still following earlier voice-leading conventions, highlights a shift toward even greater harmonic autonomy, in which the cadential six-four chord, like the opening harmonies in “Il penseroso,” is subjected to morphological alteration secured only by a well-defined, underlying tonal syntax.¹⁹ The result is not simply a change in the color of the harmony, as from major to minor, but a dramatic, seemingly jagged, change in its outward shape. An analogy could be made to painting—the technique being less like the strident color schemes of Henri Matisse’s Fauvist paintings and more like the disfiguration in Pablo Picasso’s Cubist paintings. The outlines of Matisse’s subjects remain intact, if somewhat altered or blurred. Picasso’s subjects, by contrast, experience more dramatic fissures in form, sometimes pushing them to the point of unrecognizability, despite the more precise brush strokes and more defined colors.²⁰

As a distinct category of chords derived from “common practice,” cadential six-fours offer a harmonic resource that is both directly connected with the past yet easily malleable in more modern contexts. To deconstruct cadential six-four chords into contrapuntal, harmonic, and syntactical components allows for a variety of compositional and analytical approaches that at once engage both the conventional and the innovative.²¹

Example 10 shows a reduction of Liszt’s passage, along with a voice-leading analysis and hypothetical normalization, to clarify the simple, underlying grammar of the passage while also revealing the relatively young composer’s harmonic innovation. On the surface, the progression of the closing four harmonies, iv–VI–V–i, seems unremarkable, but the inversion of the submediant to six-four alters its pre-dominant role. Strong placement of any six-four chord on the third hyperbeat of a phrase is one of the first indicators of cadential function. Hearing the pre-dominant relation of the submediant combined with the chord’s potential as a cadential six-four confuses the chord’s harmonic function. Comparing the replacement of the VI$$^{6}_{4}$$ in the progression with the conventional cadential i$$^{6}_{4}$$ (or its inversion i$$^{6}$$) reveals their commonality. The vastly different aural impression created by the original, “wrong” cadential six-four (Example 10a) as compared to the normalized diatonic version (Example 10c) makes their similarity in harmonic function and syntax even more remarkable.

If a voice-leading graph aims to show prolongation through conventional linear and harmonic connections, then displaced or seemingly detached harmonic relations present a challenge. In this case, it is not simply the chromaticism that challenges analysis; it is that traditional Stufen—scale degrees that possess inherent harmonic meaning—are displaced to sound like other Stufen. The G$$\natural$$ bass of the six-four in m. 7 most directly associates with $$\hat{3}$$ in E minor, but due to its strong metric placement, the six-four figures, and its direct association with the cadential dominant, the G$$\natural$$ functions more like a displaced $$\hat{5}$$. The dotted slur connecting the G to the B in Example 10b suggests one of two interpretations. The earlier discussion on inverted cadential six-fours might lead one to view the G as an inner voice that is placed low, effectively a i$$^{6}$$ with B displaced by G, which would make the dotted slur an arpeggiation within an underlying voice exchange. 

A competing hearing retains its six-four position, in which VI$$^{6}_{4}$$ in the score functions as a cadential six-four not by inversion of an E minor triad—with a displaced fifth—but by a transposition of the whole underlying cadential six-four: the bass G functions as a shifted or sub-posed B, together representing the same $$\hat{5}$$ Stufe. By extension, one might hear the upper voice C function as a displaced $$\hat{1}$$ (E) and the E as a displaced $$\hat{3}$$, represented by the G$$\sharp$$ in the normalized E-major ending. While smooth voice leading supports the earlier hearing as a modified inverted cadential six-four, the harmonic syntax supports the latter, in which the surface six-four is retained and decidedly cadential in function. In that latter hearing, the chord is simply a transposed, “wrong” cadential six-four.²²

With that hearing, the dotted slur in the bass from G$$\natural$$ to B in Example 10b highlights an odd connection. More than a traditional slur showing arpeggiation, the slur makes a syntactical link, like a skewed tie that retains function as $$\hat{5}$$ (as if B-to-B) behind a shift on the surface (G$$\natural$$ to B). Dotting the slur makes a more nuanced reference to Schenker’s more traditional octave transfer, which represents a preservation of function behind a change in pitch. The dotted slur here represents an obligatory correction of the misplaced cadential six-four bass to the “correct” dominant $$\hat{5}$$ to secure the cadence. 

Liszt’s enharmonic change from C$$\natural$$ ($$\flat\hat{1}$$) to B$$\sharp$$ ($$\hat{7}$$) in the opening phrase presents a similar, albeit simpler, shift in function and provides motivic basis for the extraordinary cadence.²³ Contrary to Example 10b’s dotted slur in m. 7, which connects two different pitches to the same underlying function, the earlier dotted slur in mm. 2–3 connects enharmonic pitches with different underlying functions. Working to develop further the salient C$$\natural$$ tonal problem that is introduced in m. 2 and developed throughout the excerpt, the G$$\natural$$ bass of the six-four exerts a force commonly associated with C major to pull further from a tonicization of a more normal relative major, E major (shown in Example 10c’s normalization), toward that of the minor mediant, E minor. The altered cadential six-four, therefore, serves a greater role than simply an unexpected sonority inserted into a largely conventional grammar. It intensifies a specific tonal-motivic development throughout the passage. 

David Damschroder (1990) draws historical connections between Liszt’s composition and several other works, the third movement of Beethoven’s Piano Sonata op. 26 being a more direct inspiration than Michaelangelo’s namesake sculpture. As Damschroder notes, Liszt’s harmonic innovations push beyond those of Beethoven; his “use of the ‘G-natural’ tonal area in Il penseroso makes Beethoven’s compositional behavior seem almost timid” (12). Yet, Damschroder’s analysis disregards the first strong implication of the G-natural tonal area, which is firmly established in mm. 14–15: the bass of the “wrong” cadential six-four chord in m. 7. This displaced cadential six-four chord most immediately pulls toward C major, but the tonal problem presented by the G-natural in the bass may inspire the “thinker’s” consideration of foreign tonal areas.²⁴ Neither Damschroder nor I have proposed what the innovative progression means for Liszt’s “Thinker” —not to mention the consistent placement of left-hand chords not on each downbeat but on the (displaced?) second beat of each measure—but these considerations offer initial steps. More evident in this excerpt than in the previous examples of inverted cadential six-four chords is the capacity of the normally well-defined chord to have a distorted shape while retaining syntactical function. Its preservation of function stems from three primary factors: its placement on a strong hyperbeat, its six-four figures, and its immediate resolution to the cadential dominant.

5. Displaced Cadential Six-Four Chords in Prokofiev and Shostakovich

It may seem spurious to draw connections from specific harmonic techniques of Liszt to those of Prokofiev and Shostakovich, but all three composers incorporate odd chromaticism and jarring harmonic shifts that embrace innovative harmonic treatments on one hand while maintaining direct reference to Classical harmonic syntax on the other. The first two phrases of the Gavotte from Prokofiev’s “Classical” Symphony (Example 11) contain quick, colorful harmonic shifts in tonal centers. The most striking shift is the chromatic six-four chord in the third phrase. Unlike harmonic progressions in the opening nine measures, the harmony in m. 10 defies traditional harmonic analysis.

The score shows a C$$\sharp$$-major six-four, which most immediately forms an unusual neighboring six-four between two dominants, defined by the lower-neighbor motion in the bass, A–G$$\sharp$$–A, with a potential literal analysis of V$$^{7}$$–(VII$$^{6}_{4}$$)–V$$^{7}$$. However, this chord’s function as a chromatically displaced cadential six-four is expressed by its strong accentuation and its temporal and harmonic proximity to the cadential dominant, in addition to a chromatic approach that is surprisingly conventional. 

Voice-leading analysis in Example 12 shows how this altered six-four at once embodies two normally contradictory functions, with the chord’s salient chromaticism on one hand—a kind of “flat” tonic six-four—and its highly conventional and well-defined tonal syntax on the other. The voice-leading graph respells the V$$^{7}$$ to VII$$^{6}_{4}$$ in D major to fit a more typical motion, Gr$$^{+6}$$ to cadential six-four in D$$\flat$$ major. Prokofiev’s treatment of the apparent local dominant-seventh as a Gr$$^{+6}$$ signals a cadential six-four function in the flat-tonic region. The dotted tie from the A$$\flat$$ to A$$\natural$$ in the bass represents shared dominant function of two different pitches, similar to the transformation in the Liszt example above from G to B.

A normalization that raises the six-four and its preceding “wrong” Gr$$^{+6}$$ up a half step (Example 13) reveals a more Haydnesque treatment. While the normalization does not account for the remaining weak hypermetric placement of the cadential six-four, the accentuation of the chord is strong and the underlying harmonic progression, beneath the odd surface, is quite conventional, revealing what Richard Bass (1988) might describe as underlying “shadow” structure.²⁵

An example of more extreme morphological deviation enters in Shostakovich’s Piano Prelude op. 34, No. 10 (Example 14). Similar to the Prokofiev example above, the six-four chord in m. 15 functions as a vertically displaced cadential six-four, whose syntactic clarity is obscured only by its chromatic alteration and distance from the tonic. On the surface, the chord pushes toward a brief tonicization of B$$\flat$$ minor. This foreign key relates to the underlying C$$\sharp$$ tonic as what might be labeled literally “$$\flat\flat$$vii$$^{6}_{4}$$” (Analysis 1) or interpreted as an enharmonic “$$\sharp$$vi$$^{6}_{4}$$” (Analysis 2), which recalls the tonal-motivic implication of the A$$\sharp$$ in m. 3. However, both of these labels lack sensible harmonic analysis. The obscured context reveals an absence of clear, logical harmonic relations, but the chord’s six-four figures immediately preceding a cadential dominant illuminate a logical analytical path derived from harmonic convention. Correlating the chord to a different enharmonic chord—a triple flat cadential six-four chord “$$\flat\flat\flat\text{ }$$i$$^{6}_{4}$$” (Analysis 2b)—seems quite dubious. Still, that analysis makes a direct connection to its underlying diatonic function as a cadential six-four, if largely by analogy. In addition to the literal and enharmonic interpretations, Analysis 3 hypothesizes a third interpretation of the chord, hearing the chord as a conventional six-four that lacks a root and adds embellishments, notably a B$$\flat$$ (A$$\sharp$$) to implied G$$\sharp$$ ($$\sharp$$9–8). While that hearing brings the underlying dominant function to the surface, I find it even less plausible and farther removed from the chord’s context. Analysis 3 not only lacks the essential dominant root, it also rearranges and confuses the distinct six-four figures. A more musical hearing, I think, draws more from the chord’s syntactical role than its harmonic or voice-leading connections.

As in previous examples, m. 15’s cadential six-four functions syntactically to signal a drive toward a cadence. Other characteristics support the cadential six-four hearing: (1) the bass’s wide leap down from F$$\natural$$3 to G$$\sharp$$2 in mm. 15–16 resembles a conventional octave leap, and (2) hypermetric accentuation is greater on m. 15 than m. 16 (strong–weak). Hearing the D$$\flat$$4 in the displaced cadential six-four as an enharmonic tonic C$$\sharp$$ reinforces associations with the conventional cadential six-four. At the same time, the displaced six-four chord creates an ephemeral yet deeply dramatic detour that pulls away from the tonic. Example 15 simplifies mm. 1–18 to highlight the passage’s harmonic derivation from a simple four-chord progression, i–III–V$$^{7}$$–i, along with the varying interpretations of the chromatic chord in m. 15.

The interval of transposition of Liszt’s altered cadential six-four (down a major third to VI$$^{6}_{4}$$) is greater than that of Prokofiev (down a half step to $$\flat$$I$$^{6}_{4}$$) or Shostakovich (down the equivalent of a minor third to $$\flat\flat$$vii$$^{6}_{4}$$). Yet, Liszt’s submediant harmonic relation is more closely associated with tonic. By the early nineteenth century, the submediant had already become both a favored harmonic substitute for tonic and an agent of narrative, especially with use of $$\flat$$VI in context of a major key. The cadential six-four of “Il penseroso,” therefore, crosses the syntax of a conventional cadential six-four with flat-submediant harmony that by 1839 was widely used in developments during heightened textual drama. Unlike the analysis of Prokofiev’s and Shostakovich’s chords as displaced versions of a cadential “I$$^{6}_{4}$$,” the analysis of Liszt’s harmony as a submediant VI$$^{6}_{4}$$, retains a somewhat conventional harmonic relation, thereby fusing (confusing?) the underlying dominant function (V) with surface pre-dominant harmony (VI).

In the two Russian examples, each modified cadential six-four represents a harmonic relation that reaches farther from the tonic key, despite the smaller interval of transposition. Neither Prokofiev’s nor Shostakovich’s harmonic alteration of the cadential six-four fuses pre-dominant harmony with a conventional cadential dominant. Instead, their treatments sound more abstract and more harmonically detached. As the chords distance themselves from their conventional, functional pedigree, they become more reliant on rhetoric. These modified cadential six-four chords represent specific terms inserted as clichés, with clear syntax but strange outward appearance. The mix of conventional syntax and odd vocabulary creates a quirky irony that simultaneously challenges yet satisfies tonal expectation.²⁶

6. Alternative Transformations using Inversion and Transposition

The identity of the displaced cadential six-fours in this study relies heavily on their consistent intervals above the bass. Each chord’s retention of the six-four figures through unusual transpositions from the conventional cadential six-four seems to contrast starkly with previous research into inversions of cadential six-four chords, in which voice leading places $$\hat{1}$$ or $$\hat{3}$$ in the bass. Yet, several of the examples above could be explained by taking a third path with two logical steps. This third pathway combines treatments of the two seemingly disparate alterations by first inverting the cadential six-four and then shifting one voice by step. 

The two pathways—a strict transposition of an entire six-four chord versus an inversion of the chord followed by a slight shift—not only represent different means to the same harmonic goal; a difference also lies in the mappings of the individual pitches. Compare how the two logical pathways might derive the cadential six-four from Liszt’s “Il penseroso.” In Figure 3a, the underlying conventional six-four (i$$^{6}_{4}$$ in E minor) is transposed in entirety down a third (VI$$^{6}_{4}$$), as discussed in Examples 9 and 10a. In Figure 3b, however, the underlying conventional six-four is first inverted (i$$^{6}_{4}$$ to i$$^{6}$$ in E minor) and then $$\hat{5}$$ is shifted up to ($$\flat$$)$$\hat{6}$$ to yield the apparent VI$$^{6}_{4}$$.

Table 2 reconsiders some specific examples to determine how this third path leads to each displaced cadential six-four. Similar to the complications discussed in Shostakovich’s Prelude (Example 14) of inferring missing roots and adjusting an upper voice, I find this logic overly complicated and less musical than the simpler syntactical association as vertically displaced cadential six-fours.

Example	Mapping using Inversion and Shift
Beatles, “Julia” (Example 7)	In D major, invert major cadential I$$^{6}_{4}$$ to I.     Then, shift $$\hat{1}$$ to $$\hat{7}$$ to create iii$$^{6}_{4}$$.
Brahms, Waltz (Example 8)	In E major, invert major cadential I$$^{6}_{4}$$ to I.  Then, shift $$\hat{1}$$ to $$\hat{7}$$ to create iii$$^{6}_{4}$$.
Liszt, “Il penseroso”  (Examples 9 and 10  and Figure 3)	In E minor, invert minor cadential i$$^{6}_{4}$$ to i$$^{6}$$.  Then, shift $$\hat{5}$$ to $$\hat{6}$$ to create VI$$^{6}_{4}$$.
Shostakovich, Piano Prelude  (Example 14)	In C$$\sharp$$ minor, invert a major cadential I$$^{6}_{4}$$ to I$$^{6}$$ (E$$\sharp$$ in bass).  Then, shift $$\hat{5}$$ to $$\sharp\hat{6}$$ to create $$\sharp$$vi$$^{6}_{4}$$ and spell enharmonically as $$\flat\flat$$vii$$^{6}_{4}$$.

7. Limits of Cadential Six-Four Deviations

The limits to how an altered chord can function as a cadential six-four are not easily defined, due in part to some overlap between pre-dominant function and cadential six-four function, and, perhaps moreover, due to the dependence of chord function on listener perception. The casual statement that a conventional cadential six-four “resolves to a root-position dominant” is inaccurate. Analysis tends to regard a conventional cadential six-four as a root-position dominant itself with two upper voices displaced (to the sixth and fourth). Displaced cadential six-fours in this article further shift individual voices within the dominant harmony to include other intervals or changes in the bass. Increasing displacement diminishes the apparent dominant function, and, insofar as it delays and leads to a more apparent dominant, it adopts a more pre-dominant role. The fewer of the criteria of the cadential six-four that are satisfied, the less the chord functions as a cadential six-four. The extent to which inverted, non-tonic harmonies are capable of expressing cadential six-four function remains a challenge both to theory and analysis. Complicating the issue is the dependence on the analyst’s perception and attenuation to specific elements of the music. 

Consider two examples. Example 1 showed how the second phrase in Wagner’s “Evening Star” reversed conventional, relative accentuation by placing a cadential six-four on a weak beat prior to its more strongly accented resolution. Similarly, the first phrase presents a chord closely associated with cadential six-four function. A reduction of the phrase is shown in Example 16a. Following supertonic harmony on the second hyperbeat, a chromatic $$\flat$$III$$^{6}$$ falls on the strong third hyperbeat of the first phrase and resolves to the cadential dominant in the second half (the “&”) of hyperbeat 3. The bass holds the dominant pitch ($$\hat{5}$$) from the $$\flat$$III$$^{6}$$ into V, and the melody descends from a sixth to a fifth above the bass ($$\flat\hat{3}$$–$$\hat{2}$$).

The rise in the accompaniment from the subtonic F$$\natural$$ to the leading tonic F$$\sharp$$ in the dominant counters conventional usage. This upward $$\natural$$3–$$\sharp$$3 figure above the dominant could be interpreted as a kind of inverted 4–3 intervallic motion found in the conventional cadential six-four in the approach to the leading tone, with F$$\natural$$ substituting for G. The conventional six-four in the subsequent phrase “corrects” the earlier displaced six-four simply by replacing the upward $$\natural$$3–$$\sharp$$3 inner voice motion with 4–3 and thereby strengthens their correlation.

Wagner’s cadential $$\flat$$III$$^{6}$$ here deviates from conventional cadential six-fours both in harmony, as a chromatically altered mediant, and in voice leading, with the ascending chromatic approach to the third of the dominant. This contrapuntal alteration stems not from inversion of six-four chords as seen in earlier examples, however, but from voice leading in a single inner voice. The F$$\natural$$ in the line, D–E–F$$\natural$$–F$$\sharp$$–G, makes the alteration of six-four necessary. Example 16b replaces the F$$\natural$$ with a G (D–E–G–F$$\sharp$$–G), creating a conventional cadential six-four and, as a result, demonstrating how unsatisfactory that conventional chord would have been. Wagner’s F$$\natural$$ not only forms a momentary B$$\flat$$-major triad, which makes the resolution to E$$\flat$$ ($$\flat$$VI) less distant or deceptive; the F$$\natural$$ also makes the inner voice’s line an inversion of the melody (C–B–B$$\flat$$–A–G), with the two lines converging into the G.

To include $$\flat$$III$$^{6}$$ in Example 6 as an inverted displaced cadential six-four chord, such as in the progression I–IV–$$\flat$$III$$^{6}$$–V–I, may push the connection between the chromatic chord and the conventional cadential six-four chord too far. On one hand, if cadential six-four chords can retain function either under inversion, as previous authors have shown, or under transposition of the whole chord, as I have argued, then it might follow that cadential six-four chords can retain function under both transposition and inversion. On the other hand, so many chords would fall into that category that it would stretch the concept of a cadential six-four quite thin. Not every chord that falls on a strong beat relative to the cadential dominant derives from an underlying cadential six-four. Accenting the third chords in the progressions I–IV–vii$$^{\circ7}$$/V–V–I or I–IV$$^{6}$$–Gr$$^{+6}$$–V–I, for example, highlights some characteristics shared with cadential six-four chords—all of which include $$\hat{3}$$–$$\hat{2}$$ and $$\hat{1}$$–$$\hat{7}$$ resolutions!—but to view the cadential six-four as their ancestor expands of the concept widely and perhaps inappropriately. Still, to make the comparison reveals voice-leading and syntactical similarity that transcends Roman numeral analysis.

8. Partial Closure

All of the altered cadential six-four chords above deviate in some way from convention. Their degree of deviation ranges from minor departures (as in the weak cadential six-four in Wagner’s “Evening Star” in Example 1), to inversions (as in Brahms’s Symphony in Example 3), to quite unusual harmonic alterations (as in Liszt’s “Il penseroso,” in Example 9). This last category represents the most significant challenge to the definition of the cadential six-four. Lennon’s apparent iii$$^{6}_{4}$$ and Liszt’s apparent VI$$^{6}_{4}$$ function as cadential six-fours despite their transposition and harmonic shift from dominant to other, seemingly non-dominant Stufen. Six-fours in the Prokofiev and Shostakovich examples similarly preserve underlying conventional syntax and dominant function of the cadential six-four, despite the unusual harmonic relations caused by their chromatic transpositions. The latter examples in particular illustrate the rhetorical power of the cadential six-four chord to persist as a signal of closure even as composers twist conventional tonal syntax nearly to the point of fracture.

---

References

Aldwell, Edward and Carl Schachter with Allen Cadwallader. 2011. Harmony and Voice Leading. 4^th ed. Boston: Schirmer.

BaileyShea, Matthew L. 2012. “Musical Forces and Interpretation: Some Thoughts on a Measure in Mahler.” Music Theory Online 18 (3). 

Bass, Richard. 1988. “Prokofiev’s Technique of Chromatic Displacement.” Music Analysis 7 (2): 197–214.

Beach, David. 1967. “The Functions of the Six-Four Chord in Tonal Music.” Journal of Music Theory 11 (1): 2–31.

———. 1990. “More on the Six-Four.” Journal of Music Theory 34 (2): 281–290.

———. 1992. “The Cadential Six-Four as Support for Scale-Degree Three of the Fundamental Line.” Journal of Music Theory 34 (1): 81–99. 

Brown, Matthew. 1986. “The Diatonic and the Chromatic in Schenker’s Theory of Harmonic Relations.” Journal of Music Theory 30 (1): 1–33.

Burstein, L. Poundie. 1999. “Comedy and Structure in Haydn’s Symphonies.” In Schenker Studies 2, ed. Carl Schachter and Hedi Siegel, 67–81. New York: Cambridge University Press.

Cadwallader, Allen. 1992. “More on Scale Degree Three and the Cadential Six-Four.” Journal of Music Theory 36 (1): 187–198.

Cohn, Richard. 2012. Audacious Euphony: Chromaticism and the Triad’s Second Nature. New York: Oxford University Press. 

Cutler, Timothy. 2009. “On Voice Exchanges.” Journal of Music Theory 53 (2): 191–226.

Damschroder, David. 1990. “Liszt’s Composition Lessons from Beethoven (Florence, 1838-1839): Il penseroso.” Journal of the American Liszt Society 28: 3–19. 

———. 2008. Thinking about Harmony: Historical Perspectives on Analysis. New York: Cambridge University Press.

Drabkin, William. 1996. “Schenker, the Consonant Passing Note, and the First-Movement Theme of Beethoven’s Sonata Op. 26.” Music Analysis 15 (2/3): 149–189. 

Everett, Walter. 2009. The Foundations of Rock: From “Blue Suede Shoes” to “Suite: Judy Blue Eyes.” New York: Oxford University Press. 

Fankhauser, Gabriel. 2006. “Flat Primary Triads, Harmonic Refraction, and the Harmonic Idiom of Shostakovich and Prokofiev.” In Musical Currents from the Left Coast, ed. Bruce Quaglia and Jack Boss, 202–215. Cambridge: Cambridge Scholars Publishing. 

———. 2013. “Cadential Intervention in the Finale of Shostakovich’s Piano Trio in E Minor, Op. 67.” Music Analysis 32 (2): 210–250.

Forte, Allen and Steven E. Gilbert. 1982. Introduction to Schenkerian Analysis. New York: W. W. Norton. 

———. 1982. Solutions to Introduction to Schenkerian Analysis. New York: W. W. Norton.

Gjerdingen, Robert O. 2007. Music in the Galant Style. New York: Oxford University Press. 

———. 2009. “Meyer and Music Usage.” Musica Humana 1: 197–224.

Goldenberg, Yosef. 2006. “A Musical Gesture of Growing Obstinacy.” Music Theory Online 12 (2).

Harrison, Daniel. 1994. Harmonic Function in Chromatic Music: A Renewed Dualist Theory and an Account of Its Precedents. Chicago: University of Chicago Press. 

———. 2016. Pieces of Tradition: An Analysis of Contemporary Tonal Music. New York: Oxford University Press.

Kidd, James C. 1976. “Wit and Humor and Tonal Syntax.” Current Musicology 21: 70–82.

Kirnberger, Johann Philipp. (1773) 1979. “The True Principles for the Practice of Harmony.” Translated by David W. Beach and Jürgen Thym. Journal of Music Theory 23 (2): 163–225. 

Kostka, Stephan, Dorothy Payne, and Byron Almén. 2013. Tonal Harmony, with an Introduction to Twentieth-Century Music. 7^th ed. New York: McGraw-Hill. 

Lester, Joel. 1992. “Reply to David Beach.” Journal of Music Theory 36 (1): 199–206.

Lewin, David. 2006. Studies in Music with Text. Oxford: Oxford University Press.

Lodewyckx, David. 2015. “Marpurg’s Galant Cadence in Mozart: Theoretical Perspectives, Formal Implications and Voice Leading.” Res Musica 7: 116–126. 

McCreless, Patrick. 1991. “Syntagmatics and Paradigmatics: Some Implications for the Analysis of Chromaticism in Tonal Music.” Music Theory Spectrum 13 (2): 147–78.

Meyer, Leonard B. 2000 [1992]. “Nature, Nurture, and Convention: The Cadential Six-Four Progression.” In The Spheres of Music: A Gathering of Essays, 226–261. Chicago: University of Chicago Press.

Nobile, Drew. 2016. “Harmonic Function in Rock Music: A Syntactical Approach.” Journal of Music Theory 60, 2: 149–180.

Rothstein, William. 1991. “On Implied Tones.” Music Analysis 10 (3): 289–328. 

———. 2006. “Transformation of Cadential Formulae in the Music of Corelli and his Successors.” In Essays from the Third International Schenker Symposium, ed. Allen Cadwallader, 245–278. New York: Georg Olms.

Schachter, Carl. 1980. “Rhythm and Linear Analysis: Durational Reduction.” Music Forum 5: 197–232. Reprinted in Unfoldings: Essays in Schenkerian Theory and Analysis, ed. Joseph Straus. New York: Oxford University Press, 1999: 54–78.

Stoia, Nicholas. 2015. “Triple Counterpoint and Six-Four Chords in Bach’s Sinfonia in F Minor.” Music Analysis 34 (3): 305–334. 

Temperley, David. 2017. “The Six-Four as Tonic Harmony, Tonal Emissary, and Structural Cue.” Intégral 31: 1–25.

Wason, Robert. 1985. Viennese Harmonic Theory from Albrechtsberger to Schenker and Schoenberg. Ann Arbor: UMI Research Press.

Wen, Eric. 1999. “Bass-Line Articulations of the Urlinie.” In Schenker Studies 2, ed. Carl Schachter and Hedi Siegel, 276–97. New York: Cambridge University Press.White, Christopher WM and Ian Quinn. 2018. “Chord Context and Harmonic Function in Tonal Music.” Music Theory Spectrum 40, 2: 314–335.

1. The widely used textbook by Kostka and Payne (2013), for example, introduces the cadential six-four in Chapter 9 (135) with no mention of suspensions or any “non-chord tones,” which are introduced two chapters later (175) without mention of the cadential six-four. Even Aldwell and Schachter’s 2011 textbook, which more strongly advocates linear considerations and underlying harmonic function, only mildly dissuades the vertical interpretation in its introduction to the six-four, stating that the “$$^{6}_{4}$$ should perhaps not even be labeled I$$^{6}_{4}$$” (140).
2. See Wason (1985, Chapters 2–8) and Beach (1967).
3. White and Quinn (2018) address the differing views of how harmonic function relies on content (pitches) or context (syntax).
4. My decision to use “lower case” Roman numerals in this article supports my goal to clarify localized harmony and chord quality, unlike the goal of the Stufen tradition, which uses only upper case (I, II, III, . . .) to clarify function.
5. My Figure 1 combines calculations from Meyer’s Figure 1, p. 241, and Appendix Table A3, p. 261. The general trend in Meyer’s Figure 1 appears to peak with Schubert, who perhaps surprisingly is more represented than Beethoven or Haydn. Other data may be similarly surprising, such as a greater usage found in Wagner and Wolf than in Mendelssohn, Chopin, or Schumann. Meyer’s sampling is small, of course—drawn from one edition of the Norton Scores—but a greater problem lies with his rules (239–240), which are quite restrictive and contextually insensitive. For example, he requires that the six-four chord be “preceded by a pre-dominant”—though what he means by that is not clear—and he assigns numeric values somewhat arbitrarily to yield certain statistical results. Still, his general conclusions are persuasive and would not likely be altered greatly by a more open or contextual definition.
6. This article does not address cadential six-four chords that are constructed conventionally yet behave in odd ways. Instead, focus is on those chords that appear unconventional from the outset. Matthew BaileyShea (2012), for example, discusses remarkable resolutions and questions the forces behind the rising sixth and fourth of the cadential six-four in Mahler’s Kindertotenlieder. Poundie Burstein (1999, 72–73) analyzes an odd progression leading to a cadential six-four in the first movement of Haydn’s Symphony No. 78 in C minor, in which a cadential six-four is approached chromatically from two “ungainly parallel six-fours.” The underlying, conventional progression in E$$\flat$$ major is expanded by the insertion of two passing six-fours to become, to put literally, if awkwardly, I$$^{6}$$–IV–($$\flat$$VII$$^{6}_{4}$$–$$\natural$$VII$$^{6}_{4}$$)–V$$^{6–5}_{4–3}$$–I. In Haydn’s striking approach to the cadential six-four, structural surprise and semantic surprise merge to invoke humor. For more on the relation between the two, and between wit and humor, see Kidd (1976).
7. Aldwell and Schachter (1989, 319–320) view these weak-beat, “deviant” [sic] six-four chords as resulting from an anticipation in the bass to the strong-beat dominant.
8. Doublings of the dissonant fourth above the bass are found frequently and early enough in the Baroque that they represent less of a deviation from the repertoire than a deviation from some simplified textbook examples.
9. Cutler (2009, 196, fn. 8) cites Damschroder’s discussion (2008, 43–45) on how similar arguments about these types of six-four chords were made in Koch’s Handbuch bey dem Studium der Harmonie 1811 (col 132, fig. 5).
10. Hearing the i$$^{6}$$ in m. 6 as a deceptive resolution of V$$^{7}$$/V to a modified vi, or iv$$^{6}$$/V, misses the greater syntactical role of the chord as increasing drive toward the cadence, as a modified cadential six-four, not thwarting or evading it.
11. For more on the cadential six-four’s use in rock music, see Everett (2009, 208-209) and Nobile (2016, 167–172), who shows how harmonic function depends more on syntax than on pitch content.
12. William Drabkin (1996, 152) addresses the structural role of the tonic when it falls between the subdominant and cadential dominant: I–IV–I–V–I. He cites Schenker’s graph (Der freie Satz, Fig. 22b) as showing harmonic support of a consonant passing tone, I IV (I) V I.
13. The same progression, including the vii$$^{\circ7}$$/V–I–V, is found in other popular songs, including Arlo Guthrie’s “Alice’s Restaurant Massacree” (1967) and Phish’s “Poor Heart” (1992). While one could hear the vii$$^{\circ7}$$/V as a common-tone diminished seventh resolving to tonic, I find that hearing to be overly localized and disengaged from the larger progression. “When the Saints Go Marching In” has a similar progression with a “root-position” cadential six-four:  I–V$$^{4}_{2}$$/IV–IV$$^{6}$$–iv$$^{6}$$–I–V–I.
14. To allow for an absence of the hypothetical base from a chord extends Harrison’s theory but logically elucidates the problem of the displaced cadential six-four.
15. The move from a cadential six-four to a V$$^{4}_{2}$$, as in an evaded cadence, is common enough not to be considered a deviation. Ascending fourths above the bass ($$\hat{1}$$–$$\hat{2}$$) are also not rare. David Lodewyckx (2015) examines cadential six-fours in which the fourth ascends, yielding $$\hat{1}$$–$$\hat{2}$$–$$\hat{1}$$ voice-leading motion. He credits Marpurg as the first to identify this “galant cadence.”
16. My definition of a cadential six-four chord does not require the presence of all members of the tonic triad, $$\hat{1}$$, $$\hat{3}$$, and $$\hat{5}$$. Yet, I find it notable that all examples do contain some form of $$\hat{3}$$—either diatonic, chromatic, or enharmonic. The consistency of $$\hat{3}$$ as the only scale degree found in cadential six-fours throughout this article may raise its status as an essential component, seemingly more than $$\hat{1}$$ or $$\hat{5}$$, and offers odd support for Allen Cadwallader’s (1992, 194) assertion, “The cadential six-four support for scale-degree 3 represents a perfectly logical union of harmony and counterpoint.” David Beach (1992), Cadwallader (1992), and Joel Lester (1992) offer differing views on how the cadential six-four may or may not provide harmonic support for $$\hat{3}$$. An odd exception to the scale degree tendency may be found in Night Ranger’s “When You Close Your Eyes” (1983), 3:18–3:25. The progression of the first phrase of the chorus, I$$^{6}$$–IV–vi–vii$$^{\circ}$$$$^{6}_{4}$$–IV (or IV$$^{6–5}_{4–3}$$), concludes with two harmonies that displace both a cadential six-four and the dominant, which are both shifted down a step and then corrected in the subsequent phrase. My definition excludes the double suspension above a tonic, I$$^{6–5}_{4–3}$$ (“IV$$^{6}_{4}$$” to I) as a cadential six-four, despite some similarities. Measure 8 of Mozart’s Symphony No. 41 in C major, K. 551 “Jupiter” (1788) contains an example.
17. The iii$$^{6}_{4}$$ chord has been specifically eschewed by theory instructors. In 2008, readers of the AP Theory exam made T-shirts bearing an anti-iii$$^{6}_{4}$$ emblem. This emphatic rejection was partly in jest, yet the reaction is understandable given: (1) the iii$$^{6}_{4}$$’s overuse by students in fundamentals courses who indiscriminately combine triads with triadic inversion, (2) its rareness in practice, and (3) its functional ambiguity, sharing two pitches with both tonic and dominant triads.
18. I interpret the C$$\sharp$$ ($$\hat{7}$$) presented on the downbeat as the primary bass note, which continues the pattern from the opening chords and yields the odd iii$$^{6}_{4}$$. To interpret the subsequent F$$\sharp$$ in the same measure not as an embellishing arpeggiation of the bass but as more structural would place the mediant in root position. While this analysis would make the chord not a six-four but a five-three (root-position) chord, the chord’s syntactical function could still be connected directly to that of a cadential six-four.
19. Figure 1 (based on Meyer, 2000/1992, 241) shows Liszt’s usage of the cadential six-four to be remarkably low, signifying the composer’s personal attraction to innovative harmony and more generally supporting a general decline of the chord’s usage.
20. Compare, for example, Fauvist colors in Henri Matisse’s 1905 The Green Line [Portrait of Madame Matisse] to Cubist disfiguration in Pablo Picasso’s 1937 Weeping Woman.
21. Harrison (2016, 10) makes a similar point, that innovative approaches to tonal harmony modify some components of conventional “common-practice harmony,” which he describes as an “interlocked system of components that includes conventions of counterpoint and voice leading, rhythm, vertical sonority, and normative syntax.”
22. A third underlying hearing could retain the upper voices (C, E, and G) above an implied bass note B to yield a dominant with $$\flat$$9, $$\flat$$6–5, and 4–3 figuration. That interpretation would hear the dominant function but not the cadential six-four character.
23. Richard Cohn (2012) explores enharmonicism in this passage as it relates to the concepts double syntax, double-agent complex (from David Lewin), and Mehrdeutigkeit.
24. Damschroder’s voice-leading graph, his Example 6b, shares much in common with mine but more immediately relegates the bass G$$\natural$$ to an inner voice, corresponding to an inverted cadential six-four.
25. In 2006, I made an analogy of this harmonic shift to the refraction of light, in which the image of the refracted object—the enclosed passage in Example 12—appears distorted from a hypothetical image of an unrefracted object, as proposed in Example 13. Unlike a shadow, a refracted image represents the “true” object only with an apparent shift.
26. The cadence in final measure of Prokofiev’s March from The Love for Three Oranges op. 33 (Act 2, Rehearsal 173) similarly blends traditional syntax with a modified cadential six-four. The chords D/A–G/F–C on the surface may appear literally as V$$^{6}_{4}$$/V–V$$^{4}_{2}$$–I in C major but function syntactically and more deeply as alterations of a conventional cadential six-four progression.